Diamonds from the Deep: How Old Are Diamonds? Are They Forever?

Karen V. Smit and Steven B. Shirey

Age Is Important

The age of something is fundamental. Humans, animals, wine, cars, and antiques are viewed and understood in the context of their age. So it is with rocks and minerals. A geologist needs to know the age of rocks to construct the geologic history of an area. In the field, relative ages can be determined by cross-cutting relationships (the younger rock “cuts” across the older rock) or superposition (the younger rock overlies the older rock). To determine the absolute ages of rocks and minerals such as diamond, scientists measure naturally occurring radioactively decaying elements. Absolute ages are free of any knowledge of relative age relations to any other geological material. This is known as the science of geochronology.

Early diamond hunters in South Africa cared little about absolute age because diamonds were found as alluvial material in riverbeds and beaches, and only where they could be found mattered. Once diamonds were discovered to be hosted in kimberlite, absolute diamond ages became important in exploring for more diamonds, as well as answering the key questions about how they form:

• Are diamonds the same age as the rock (kimberlite) in which they are found?
• Did they crystallize from the kimberlite or were they picked up from country rock during volcanic eruption?
• What are the ages of kimberlites? What are the ages of the diamonds?
• Can one mine have diamonds with multiple ages?
• Are diamond-forming events a result of specific larger-scale geologic processes?

A benefit of this quest has been to show that the oldest mineral sample you can obtain and wear as jewelry is a diamond that is often three billion years or older—this is almost three-fourths of the earth’s age. This attribute will never be matched in any synthetic diamond.

Radioactive Decay and Mineral Age

The most robust way to determine the absolute age of any mineral or rock is through radiogenic isotope analyses. Long-lived radioactively decaying elements such as uranium, samarium, rhenium, and rubidium have one or more isotopes that spontaneously decay (known as a “parent”) to the isotope of another element (or “daughter”) at a constant average rate (box A). Modern mass spectrometers can measure infinitesimally small differences in the parent and daughter isotopes that have been trapped in minerals by measuring isotopic ratios. From this data and by knowing the isotopic decay rate, the mineral’s age can be calculated.

BOX A: Isotopes and Radioactive Decay

To understand basic radioactive decay, some atomic-level definitions are necessary. Each element of the periodic table is composed of atoms that have the same number of protons in the nucleus and electrons in a shell surrounding the nucleus. However, the number of neutrons in the nucleus may vary, and when it does, it will be a different isotope of the same element. In other words, an isotope of an element is simply the same element with a different number of neutrons. Radioactive decay is a nuclear process that affects the number of protons and neutrons and thus forces us to think in terms of isotopes. As shown in figure A-1, there are three types of different natural radioactive decays: alpha (α), beta (β), and gamma (γ). For dating of geological materials, we are concerned only with alpha and beta—both of which involve the change of an isotope of one element, the parent isotope, into an isotope of another element, the daughter isotope. Radioactive decay of parent to daughter isotopes (figure A-2) occurs randomly, but for many atoms the average decay rates over time are very constant. This constant, known as a decay constant, can be measured and is specific for each decay scheme. The half-life refers to the time it takes for half the amount of parent isotope to decay to its daughter isotope and is an inverse function of the decay constant. Isotope systems with very long half-lives such as uranium-lead, samarium-neodymium, lutetium-hafnium, rubidium-strontium, and rhenium-osmium are employed for geological materials that can have ages anywhere from 4.6 billion years ago (the age of the earth) to the present day.

Figure A-1. Three different types of radioactive decay, and the atomic models for their radiation (from Breeding et al., 2018).           Figure A-2. Common parent-daughter isotope systems that have sufficiently long half-lives to be used for dating of geological materials. The age of the earth was determined using the U-Pb isotope system. The majority of worldwide diamonds are dated by measuring Re-Os isotopes in sulfide inclusions.

The assumption for isotopic dating is that each mineral behaves as a tiny closed system. The mineral starts with a known quantity of the parent and the accumulation of the daughter is measurable because original amounts of the daughter isotope are insignificant, and what accumulates cannot escape from the mineral structure. What occurs in nature is the equivalent of an hourglass where the mineral is the glass and the decaying parent and accumulating daughter are the sand. The neck in the glass that controls the rate at which the sand passes is the radioactive decay rate. As long as the mineral has remained a closed system from the time of its formation to its analysis in the lab today, an absolute age of the crystal is obtained.

In theory all minerals could be dated this way, but in practice only a small number of minerals can actually be dated. Limitations are due to low abundances of radioactive elements in a mineral’s structure, a decay rate of the parent isotope that is too slow, poor retention of the daughter isotope under certain geological conditions, and inadequate analytical sensitivity.

If Diamonds Cannot Be Dated Directly, How Can They Be Dated at All?

Although diamond is composed primarily of carbon, it cannot be carbon-dated since the half-life of carbon is too short (atmospheric ¹⁴C decays to ¹⁴N with a half-life of only 5,700 years) to be useful for any geological material such as diamond that typically has ages on the order of millions to billions of years. Diamonds also do not contain sufficient amounts of any of the radioactive elements mentioned in box A. Instead, geochronologists use mineral inclusions such as iron sulfide, clinopyroxene, and garnet that are trapped within the diamond and contain sufficient Re-Os, Rb-Sr, and Sm-Nd to determine diamond ages. The simplest assumption is that the obtained age indicates how long the inclusion was trapped in the diamond, and therefore gives the time of diamond formation.

It should be apparent now that ages cannot be determined for diamonds that do not contain large enough or any mineral inclusions—in other words, the vast majority of gem-quality diamonds. Among members of the gem trade, mineral inclusions trapped within diamonds are not normally considered a desirable feature. However, these rare mineral inclusions are extremely valuable scientifically as they are the only direct samples that geologists have to study Earth at depth; inclusions in some diamonds have been documented to originate from more than 660 km depths. And inclusions are the only way to determine a diamond age!

Unfortunately, diamond dating is a destructive technique. Mineral inclusions have to be broken out of the diamond so that they can be characterized, dissolved, and analyzed for their isotopic composition. It is not possible to obtain a diamond formation age from a single inclusion. From an isochron diagram (box B), it should be clear that isotopic compositions for multiple inclusions are needed, from which a slope is determined and an age calculated. Most often, multiple inclusions of a similar paragenesis (or host rock type) are analyzed to find a formation age for a suite of diamonds from the same locality. Occasionally, scientists get lucky and are able to obtain multiple inclusions from a single diamond. In this case, the diamond’s formation age can be obtained or even a long-lived growth history may be revealed.

BOX B: The Isochron Diagram

A graphical method of evaluating the data produced by the mass spectrometer is needed to determine an age. In this example of an isochron diagram (figure B-1), the analyses of three different minerals (blue circles) have been plotted. The x-axis on the diagram displays the ratio of the radioactively decaying isotope to a non-decaying, stable isotope of the daughter. A ratio is necessary on this diagram because mass spectrometers measure ratios well and we need to know how much parent isotope is available for decay relative to the daughter. The minerals with higher amounts of parent plot further to the right. The higher the content of parent, the more daughter is produced. These minerals plot at a higher position on the y-axis, which is the ratio of the radiogenic daughter to the same stable daughter isotope as on the x-axis. Radioactive decay (red dashed arrows) is a transformation of a single parent isotope of one element to a single daughter isotope of another element. This radioactive decay process preserves the slope relationship that will be generated by different amounts of parent (relative to daughter) in the different minerals. By using the slope of a line fitted through the minerals (blue line) and the independently determined decay rate (accepted by the scientific community as a constant), the true or absolute age may be calculated. In principle this may seem complicated, but in reality the calculation is quite simple. The chief difficulties are preparing the samples in the chemistry lab for analysis and understanding the geological relationship between different minerals. For illustration purposes only, the “original” positions of the minerals are shown at the time they (re)crystallized (gray circles).

Figure B-1. The isochron diagram shown in this figure is a typical graphical way of expressing the calculation of absolute age. Minerals measured at present in the mass spectrometer fall on a line whose slope correlates directly with age.

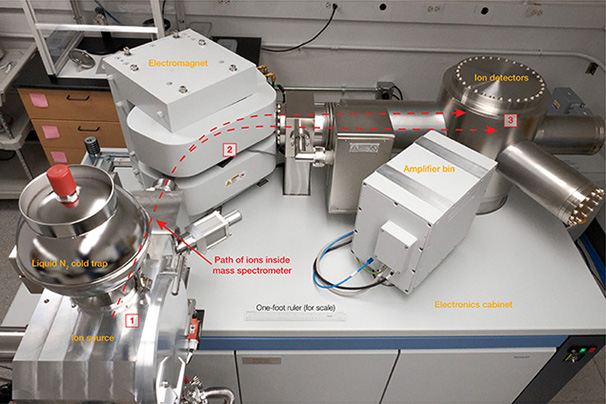
Once the mineral has been released from its diamond host, parent and daughter elements must be removed from the mineral and purified. Since minerals are solid, removal and purification of the parent and daughter elements first requires dissolving the mineral in very strong acid solutions (such as mixtures of chromic, sulfuric, hydrochloric, nitric, and hydrofluoric acids). Once the mineral is dissolved, miniaturized purification methods—using essentially the same type of polystyrene resin beads that are found in household water filters—are applied to separate out extremely pure parent and daughter elements. But since elements are a combination of both radioactive isotopes and so-called stable isotopes that do not decay, a further step is needed to separate out the radioactive isotopes for analyses. To separate and measure them, we turn to the mass spectrometer (box C). In this final step, the dried-down salt of the element of interest is placed onto a metallic filament that is then placed into the mass spectrometer to be slowly heated and ionized. The ions are accelerated through a curved magnetic field, and each isotope is then counted separately in a specialized detector. The detectors in a modern mass spectrometer are sensitive enough to count each and every ion that passes through its magnetic field.

BOX C: The Mass Spectrometer

The mass spectrometer is the final analytical stage for determining an absolute age. Mass spectrometers like the one shown in figure C-1 are found all over the world and used chiefly in the nuclear industry and geology. The dried-down salt of the element of interest (e.g. lead, osmium, or strontium) is placed onto a metallic filament and heated to produce ions in the ion source at position 1. High voltage of around 10,000 volts accelerates the ions through the magnetic field created by the electromagnet at position 2. The magnetic field forces the ions into a curved trajectory; the lighter isotopes curve more (inside curve) and the heavier isotopes curve less (outside curve). In this way, the different isotopes can be separated (as shown by the diverging ion paths) and detected individually at position 3. Although only two paths are shown for clarity, this mass spectrometer can produce and detect up to nine different isotope beams separately. Other mass spectrometers can produce and detect more than 16 isotope beams at one time! Below the table surface is an electronics cabinet containing pumps, power supplies, circuit boards, an on-board data acquisition computer, and motor drives. Not shown is the instrument control computer that allows the scientist to control the progress of the measurement. Up to 21 samples can be loaded into the mass spectrometer at one time, and modern internet connectivity allows operation from any location.

Figure C-1. The Thermo Scientific Triton Plus mass spectrometer used at the Carnegie Institution for Science. Photo by Steven Shirey.

The History of Diamond Age Determination

The first diamond ages were determined by Professor Stephen Richardson of the University of Cape Town when he was a graduate student working at the Massachusetts Institute of Technology in the early 1980s. Using samples from the Kimberley mines in South Africa (figure 1; Richardson et al., 1984), single grains of garnet or clinopyroxene from gem-quality monocrystalline diamonds needed to be grouped together with other identical grains—each broken from its own diamond host—for just one chemical analysis. Grain grouping had to be done to obtain sufficient Sm, Nd, Rb, and Sr to analyze. This technique was cutting-edge during its time, and the resulting study definitively showed that diamonds are much older than their host kimberlite. Some of the Kimberley diamonds were more than three billion years old, lending truth to the De Beers slogan “A diamond is forever.” Richardson and coauthors subsequently provided age constraints for many diamond suites from South Africa, Botswana, Australia, and Russia (e.g., Richardson, 1986; Richardson et al., 1990; Richardson and Harris, 1997) and demonstrated that there were multiple generations of diamond between one and two billion years ago in the southern African mantle.

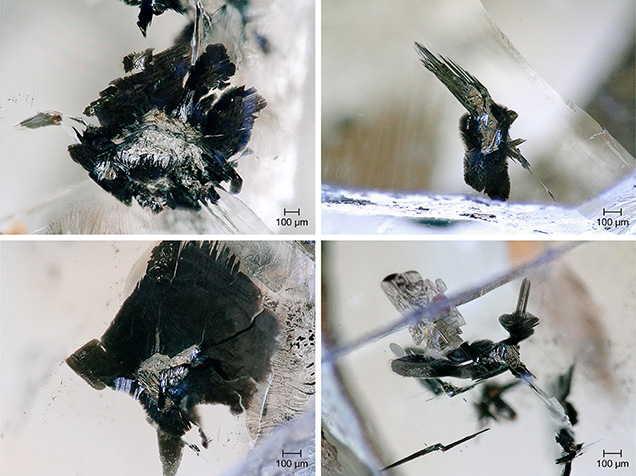
In the mid-1990s, Professor Graham Pearson and Dr. Steven Shirey working at the Carnegie Institution for Science miniaturized and modified the Re-Os analytical methods used on whole-rock samples so that single sulfide inclusions (typically between 2 and 10 μg but also larger, up to 30 μg; figure 2) could be analyzed rather than having to group all the inclusions together. Since the initial Re-Os study on Koffiefontein sulfide-bearing diamonds (Pearson et al., 1998), this technique has become the most widely used method to determine global diamond ages (e.g., Richardson et al., 2001; Westerlund et al., 2006; Aulbach et al., 2008; Richardson and Shirey, 2008; Aulbach et al., 2009; Smit et al., 2010; Wiggers de Vries et al., 2013; Smit et al., 2016; Aulbach et al., 2018).

Recent analytical advancements (namely even more sensitive and accurate detectors in mass spectrometry) have made it possible to measure Sm, Nd, Rb, and Sr at the extremely low concentrations in which they occur in single garnet and clinopyroxene inclusions. Researchers working with Professor Gareth Davies at the Vrije University in Amsterdam have now started using this technique to study diamond ages from Botswana and South Africa (Timmerman et al., 2017; Koornneef et al., 2017). This technique will certainly become as widely used as the Re-Os isotope system in sulfide inclusions, and for diamond suites where both silicates and sulfides occur, the two techniques can be used in conjunction.

A new method emerging from work at the Australian National University that shows promise in this evolving field is the dating of fluid-rich fibrous diamonds using the U-Th/He isotope system. In this system, trapped micro-fluids contain sufficient ^235,238U and ²³²Th that decay over time to ⁴He (Timmerman et al., 2019). Where mineral inclusions are used to date monocrystalline gem-quality diamonds, fluid inclusions can be used to date a separate suite of fibrous diamonds and give important constraints on whether they formed in association with kimberlite eruption.

The Relationship of Mineral Inclusions to the Diamond

A long-standing requirement for diamond dating has been that the inclusions and the diamond co-crystallized from the same diamond-source fluid. In other words, that there is a syngenetic relationship between diamond and inclusion, as opposed to the diamond incorporating a preexisting mineral grain. The assumption of a syngenetic relationship has traditionally been based on textures observed in diamond inclusions that show that the diamond’s cuboctahedral morphology has been imposed (e.g., Harris, 1968; Harris and Gurney, 1979). Epitaxial crystallographic relationships between inclusions and diamond (e.g., Mitchell and Giardini, 1953) can also be a key indicator of this syngenetic link.

However, there is now increasing evidence that inclusions in diamonds can also be preexisting rather than syngenetic (Thomassot et al., 2009; Smit et al., 2016; Milani et al., 2016; Nestola et al., 2017)—a fact that explains some long-standing compositional features of inclusions. In fact, some inclusions could have a syngenetic interface with diamond even if crystallographic evidence suggests the inclusion was preexisting (Agrosi et al., 2016).

In practice, a lack of syngenecity is not necessarily a problem for dating studies based on inclusions, since the isotopes in these older minerals are often re-equilibrated, or reset, at the time of diamond growth so that they still yield diamond formation ages (e.g., Smit et al., 2016; Smit et al., 2019). Even if isotopic equilibration did not take place, the errors on diamond ages are often sufficiently large (typically on the order of >100–200 Ma or sometimes greater; Westerlund et al., 2006; Aulbach et al., 2008, 2009; Smit et al., 2010) that if an inclusion was preexisting, it might still have formed within error of the determined age. While such large errors may seem horribly imprecise, they are more than adequate for diamond geology and in fact represent huge advances: separating out different diamond-forming events, relating the diamond-forming events to geological processes, and correlating diamond-forming events between different mines.

What Have Diamond Ages Taught Us?

From isotope studies of inclusions in diamonds, we now know that diamonds have formed nearly throughout Earth’s history (figure 3). The oldest dated examples, the 3.5–3.3 billion-year-old Diavik and Ekati diamonds, were forming prior to the rise of oxygen in the earth’s atmosphere (2.5 to 2.3 billion years ago). All diamonds that have been dated so far were formed even before the dinosaurs went extinct 65 million years ago. We have no reason to believe that diamonds are not still forming right now deep in the earth’s mantle. However, since they form so deep and do not survive the oxidizing conditions of common basaltic volcanism, we cannot access them until rare kimberlite eruptions bring them to the surface.

Aside from simply knowing a diamond’s age for curiosity’s sake, diamond ages have proven to be extremely valuable tools for geologists studying the deep earth. Diamond ages have told us that diamonds are much, much older than kimberlites. They did not crystallize in the kimberlite but are only passengers riding a volcanic eruption to the surface.

Diamond ages have changed our thinking about the association of diamonds with ancient continental mantle keels. Contrary to traditional thinking, diamond ages have shown that they can also form in active tectonic regions around cratons. Incorporation of such areas has expanded diamond exploration targets and will contribute to finding new diamondiferous kimberlites.

Diamond ages have also allowed us to link their formation to known plate tectonic processes. We now know that subduction of hydrated oceanic slabs is how most of these diamond-forming fluids are supplied into the deep earth. Diamond ages have placed important time constraints on the recycling of fluid mobile compounds and elements such as water, carbon, nitrogen, boron, and sulfur into the deep earth. There is no other way to trace these so deeply and as far back in time.

Through age determinations, we have learned that diamonds are not only forever but they are forever revealing new secrets about our dynamic planet.