Observations of Oval-, Pear-, and Marquise-Shaped Diamonds: Implications for Fancy Cut Grading

ABSTRACT

A cut grading system useful at all levels of the diamond trade must encompass the visual appeal of polished diamonds. This paper presents results from observations of oval-, pear-, and marquise-shaped diamonds for face-up appearance and outline appeal. In a controlled environment, an international cross section of diamond buyers, sellers, cutters, and appraisers observed sets of research diamonds in each shape; internal observation teams also examined diamonds submitted to GIA for grading services. Observers consistently disliked diamonds with prominent windowing but showed a broader range of opinions regarding the “bow tie” and “crushed ice” patterns typically found in these rounded fancy shapes. The distribution of opinions indicates significant differences in both regional preferences and personal taste for certain outlines and appearance patterns. Virtual facet maps, calculated from the three-dimensional wireframe file of a polished diamond, provide a quantitative visualization of these patterns. The geometric complexity of fancy shapes with multiple faceting arrangements requires the use of those same 3D files for assessing or predicting light behavior in a polished diamond.

GIA recognizes more than 40 distinct categories of fancy-shaped diamond,¹ each with unique characteristics and appearance qualities and continuously evolving facet arrangement variations. Brightness and fire remain important concepts for grading cut in fancy shapes, as they are for standard round brilliants. However, fancy-shaped diamond appearance patterns (figure 1) include aspects not displayed by the more constrained round brilliant at any proportion combination. Fancy shapes show more varied and pronounced changes to that patterning with motion than rounds, changes that produce scintillation—sparkle and liveliness. Further, rounded fancy shapes are cut with a number of outline variations beyond the length-to-width ratio (L:W), affecting both the face-up appearance and overall appeal of a diamond. It is clear that the cut grading methods applied to round brilliants cannot be simply transferred to fancy-shaped diamonds. Each fancy shape merits its own tailored approach to cut quality grading, taking into account its unique set of visual attributes.

A useful cut grading system should make visual sense. For round brilliants, Moses et al. (2004) found that most people agreed on the qualities that diminished a diamond’s appearance. Some of these qualities apply equally well to fancy shapes. For example, all observers dislike dark or dull areas that reduce the overall brightness of the stone and mar its attractiveness. As these areas become more pronounced, they detract even further. Most observers expect to see some fire—dispersed light with sufficient intensity and covering large enough areas to be noticeable. People generally prefer distinct and orderly contrast patterns that are not overly blocky or highly fragmented. However, the diverse range of patterns found in fancy shapes tap into deeper levels of individual preference or personal taste and aesthetics that extend beyond the basic appearance requirements for a faceted diamond. As some patterns are shape-specific, these taste factors also extend to a preference for a particular outline. Acknowledging varying taste factors² within a cut grading system is challenging for each fancy shape. Assessing overlap and differences among observers looking at a set of diamonds with different appearances is an essential first step for building such a system.

¹GIA uses the term fancy shape to include non-round outlines as well as facet arrangements of round shapes other than the 57/58-facet standard round.

²During this study, it became obvious that observers who prefer a “hearts and arrows” appearance in round diamonds caused by observer reflection tend to dislike the same reflection pattern when it occurs in oval, pear, and marquise shapes, resulting in what the jewelry trade calls a “bow tie.”

BOX A: DESCRIBING THREE COMMON CURVED OUTLINES

The word oval means “egg-shaped” (from the Latin ovus, meaning egg), but oval gemstones are more symmetrical and elliptical in outline than most eggs. Most oval-outlined diamonds are slightly broader at the shoulders than an ellipse but more rounded than cushion shapes (figure A-1). Ovals that are flatter at the shoulders than an ellipse are often called “movals” by the trade, as the outline becomes closer to a marquise; ovals that are quite squat and almost round are called “rovals.”

The outline of a pear-shaped diamond is rounded at one end (the head) and tapered to a point at the opposite end. A long, narrow pear is sometimes called a “pendeloque.” The head shape can vary from semicircular to broad. The wings (see again figure A-1) can be plump or flat, and the angle of the point varies following the influence of the wing shape.

A marquise outline is an elongated elliptical shape with curved sides and two pointed ends. This is also called a “navette,” from the Latin for “ship,” because its face-up outline resembles a simple boat. Marquise shapes are fashioned with a range of point angles and wings that are either plump or flat (see again figure A-1).

Oval-shaped diamonds are commonly designed with L:W ranging from 1.2 to 1.7 (Blodgett et al., 2011). Pear shapes usually have L:W from 1.3 to 1.8. Marquise diamonds are typically longer with L:W from 1.5 to 2.5. All three shapes are usually cut with brilliant faceting, with many choices of facet arrangement (figure A-2). Many ovals have eight bezels matched with four, six, or eight pavilion mains, but some have ten or twelve bezels. Additional facet rows on the pavilion further add to the variety. Pear shapes typically have seven or eight bezels matched with anywhere from three to nine pavilion mains. (The single symmetry axis supports the odd-numbered main arrangements.) Pear and marquise shapes may have “French tips,” in which several facets replace the bezel at a point. Marquise diamonds typically have six or eight bezels matched with four, six, or eight mains, and other more complicated pavilion arrangements. All three shapes can be fashioned with step faceting or as mixed cuts (step-cut crown and brilliant-style pavilion, or vice versa), but such diamonds are unusual and were not included in this investigation.

BOX B: PARAMETER DESCRIPTIONS FOR OUTLINE AND PROPORTIONS

Many parameters are required to describe just one fully symmetrical faceting arrangement with four pavilion mains in these three fancy shapes. The geometric parameters shown in figures B-1 and B-2 relate to gemological ways of describing the outline and proportions.

Curvature and L:W combine to describe whether an oval has large (broad) or slack (flatter) shoulders; adding in the point angle describes whether the wings of a marquise are plump or flat (see again box A). Girdle thickness indicates the separation of the crown from the pavilion, while the sets of girdle vertices determine the outline positioning of the bezels and pavilion mains. The table vertex parameters describe the size and shape of the table and further constrain the position of the bezels. The distance parameter for the interior crown meetpoints describes the star lengths, and their position parameter affects the shapes of the bezel facets and stars. Given these constraints, a single crown angle value resolves the angles for the rest of the crown facets.

Similarly, the interior meetpoints in the pavilion describe the lower half facet lengths. With four pavilion mains, these meetpoints must lie along the length and width axes of oval and marquise shapes (see again figure B-1) and along the length axis of the pear (see again figure B-2). Since the girdle vertices constrain pavilion main positioning, only one pavilion angle is needed to solve for the others.

For the pear shape, the rounded portion and the pointed portion are mathematically independent. As illustrated in figure B-2, this shape needs two parameters for curvature and separate parameters for L:W of the head and L:W of the point. The pear needs a parameter for the displacement of the culet along the vertical axis because the culet is not constrained to lie at the width position. Eight table vertex parameters describe the length, width, and shape of a pear’s table, and another eight crown meetpoints describe the star lengths and the shape of the bezel facets. Then, defining a single crown angle constrains the rest of the angles. The seven pairs of girdle vertices set the positions of the four pavilion mains and the lower half facet junctions, and the pavilion meetpoints constrain the lower half lengths and width of the pavilion mains. With these other parameters, a single pavilion angle constrains the rest of the angles.

This article will discuss observations of oval, pear, and marquise shapes (described in box A). These three shapes share some geometric elements with the round brilliant. An oval is an elongated circle that can be modeled by a mathematical ellipse. A classic marquise is the intersection of two circular arcs (Watermeyer, 1980). A pear can be modeled by the combination of a marquise and an oval. Gem manufacturers utilize such mathematical models to help polish smooth and symmetrical outlines (Watermeyer, 1980). The similarities promote the reworking of concepts and tools from the round to these shapes, while the differences lead to additional factors to explore for the evaluation of cut. Oval, pear, and marquise shapes are found across a range of L:W, fashioned in a number of typical brilliant faceting arrangements and outline variations. Many parameters are required to describe the combination of variations of outline curvature, faceting arrangement, and proportions for those facets in each shape.

Variation among just six parameters for the round brilliant led to more than 38 million proportion combinations to encompass the proportions typically encountered in diamond grading. Fancy shapes require many more parameters, leading to an astounding number of possible combinations. For instance, a concise geometric description of one symmetrical faceting arrangement for an oval—eight bezels, four pavilion mains, and no painting or digging out (Blodgett et al., 2005)—requires 18 parameters, three times more than the round brilliant (box B). An oval arrangement with eight pavilion mains that includes painting variation increases the number of parameters to 28 (Conant, 2024). A symmetrical marquise with four pavilion mains requires all the parameters for the oval, plus the angle at the points, for a total of 19 parameters. Describing a pear shape with four mains requires 35 parameters because the curvature of the rounded portion and the pointed portion of the outline are independent, and the facet positions are less constrained. With eight pavilion mains and painting variations, the pear shape parameters increase to 57 (Conant, 2024). The much greater number of parameters for fancy shapes precludes any kind of grade prediction based on rounded proportion combinations. Any predictive cut grading for fancy shapes must be based on a 3D representation of the diamond, such as the wireframe files produced by non-contact measuring devices.

BACKGROUND

For many decades, the trade has debated which ranges of L:W, table percentage, and total depth percentage produce attractive fancy-shaped diamonds. As trade interest in proportion-based cut grading increased through the 1990s and into the 2000s, various ranges were promoted for some fancy shapes, along with parameter range charts for round brilliants. In 2005, the American Gem Society (AGS) unveiled its use of a three-dimensional ray-tracing model for light performance evaluation and produced corresponding ASET images (Sasian et al., 2007; Gilbertson, 2013). As research continued, some fancy-shaped diamonds were added to their Light Performance system. However, this approach required continual research to update reference standards for grading additional faceting arrangements among supported shapes. In support of the industry, manufacturers and retailers had access to proprietary software that could provide an estimated grade for a diamond by utilizing the 3D model created from a non-contact measuring device.

Octonus, in collaboration with Lexus Group, has applied various metrics for appearance aspects to fancy shapes; these metrics were originally developed for standard round brilliants (https://www.octonus.com/projects; see also https://legacy.octonus.com/oct/products/3dcalc/standard/diamcalc_2-0.phtml). This technology addresses several aspects of human vision, notably the effects of stereovision and the contribution of contrast to the perception of brilliance (Sivovolenko et al., 2013). Established by Lexus SoftMac in 2016, the Cutwise web platform is the chief source for this assessment of cut (https://cutwise.com). It accepts a wireframe file or imagery from the DiBox equipment suite and produces various images and videos along with an evaluation by the Cutwise metrics collection.

In the autumn of 2022, the International Gemological Institute (IGI) announced a cut grading service for nine fancy shapes (International Gemological Institute, 2022). They established proportion ranges “historically observed to produce positive beauty,” hinted at additional shape-specific restrictions (e.g., bow tie factors), and left the final cut grade to a gemologist’s visual evaluation of “how effectively the diamond reflects light back to the viewer” (International Gemological Institute, n.d.).

BOX C: WIREFRAME FIDELITY

Computer-driven gemstone measuring devices (such as the Lexus Helium system or the Sarine DiaVision) capture the skin of a polished diamond and construct a wireframe file—a mathematical representation of the vertices of the diamond and how they connect into facets. When this wireframe closely matches the measured diamond, it provides a model geometry for tracing how light moves through that stone (figure C-1). However, many fancy-shaped diamonds produce a wireframe file with significant differences from the actual stone. Shallow crown depth, shallow pavilion depth, and faceting designs that lead to small interfacial angles all make it more difficult for such measuring systems to render all the facets as seen on the diamond. Additional information about facet edges from a crown or pavilion view of the diamond is needed to correct such deficiencies. Ray-tracing light through a badly rendered wireframe may produce results that have little to do with the diamond’s actual appearance.

The visual appeal of polished diamonds is a critical factor for a fancy cut grading system. A useful system must also support the design and cutting of attractive fancy-shaped diamonds for traditional choices, as well as for new faceting arrangements, proportion combinations, and outline variations. Accurate cut grade estimation during rough planning allows manufacturers to make sound business decisions, and the large number of fancy-shape parameters (see again box B) requires wireframe files for the analysis that leads to a grade evaluation. However, a wireframe-based prediction is only as good as the fidelity of the wireframe (i.e., the degree to which it represents the diamond). Wireframes that do not closely match the stone’s faceting produce anomalous results, as shown in box C.

While developing the GIA cut grading system for the round brilliant, we found that the face-up pattern—the distribution of bright and dark elements—was as important as the metrics for light return and fire in evaluating overall diamond appearance (Moses et al., 2004). This importance arises from the nature of human vision, which is more sensitive to the intensity and distribution of contrasting elements in a visual display than it is to the quantitative amount of light return (Yaguchi, 1987; Kingdom, 2003; Heeger, 2006; Gilbertson, 2013). Thus, the visual impression of brightness is largely drawn from these face-up patterns. Overall diamond appearance also includes how these patterns change with the motion of the diamond (or viewer, or light source), creating scintillation. Rounded fancy shapes display two pattern aspects of particular interest: “bow tie” and “crushed ice.”

The elongation inherent to oval, pear, and marquise shapes tends to produce a bow tie as part of the visual pattern for most choices of brilliant faceting arrangement and wide ranges of proportions (figure 2). This area of dark contrast seen through the table across the width of the stone can vary from slight to thick, and it may be made up of disjointed segments or form a continuous dark bar. Some bow ties are persistent, while others brighten during motion of the diamond. The choice of faceting arrangement and faceting proportions can further accentuate or diminish bow ties.

These shapes generally produce areas with many small virtual facets (Sasian et al., 2007) often described by the trade as crushed ice (again, see figure 2). The size distribution of virtual facets in such “busy” areas can vary. Small virtual facets may dominate some diamonds, while others may show a broad range of virtual facet sizes. Crushed ice can also show differing amounts of contrast, from distinct and bright to muddled and gray. For most proportion combinations, all three shapes tend to show regions with this pattern, especially at points; with some facet arrangements, crushed ice dominates the pattern across the entire stone.

MATERIALS AND METHODS

Despite the daunting task of evaluating billions of possible fancy-shape combinations that manufacturers can produce, ultimately human observations provide the essential ground truth for tuning any predictive system. Both people and actual diamonds are required to collect human observations of diamond appearance. Using consistent lighting and observing conditions and the same set of diamonds for all observers provides the basis for exploring the variance (range of differences) among them. Quantitative data can be drawn from such observations by having observers perform specific tasks, such as putting a set of stones in order from best to worst appearance or evaluating a group of stones in pairs until all have been compared.

The authors assembled an initial reference set of fancy-shaped diamonds containing 21 ovals, 16 pear shapes, and 16 marquise shapes, including examples with different numbers of pavilion mains in each shape (table 1). Each research diamond (RD) is designated by a unique number. For testing, we used 12 of each shape, in two sets of six stones for several kinds of observations, noted in table 1 as set A or B (figure 3). Since 2023, more stones have been added to the reference collection as research continues to include additional faceting arrangements, outline variations, and particular pattern elements.

A mobile lighting and viewing environment was developed to gather observations from trade members in six worldwide locations and from several teams of internal GIA observers. The chosen lighting and observing conditions were documented and standardized to ensure that each observing station was identical and observers followed a common procedure. The station consisted of a GIA DiamondDock, with GIA fluorescent bulbs set in the center position of the lighting cabinet and a diffusing plate below. Observation stones were set in neutral gray plastic trays on the floor of the DiamondDock. Observers held the tray close to the bottom of the viewing environment (with part of their hands touching the base) and centered 10 to 12 in. (25 to 30 cm) under the light source and 12 to 18 in. (30 to 45 cm) from their eyes. The tray with the stone or stones was gently rocked to add motion for some observations. For recent internal observations (described below), each observer wore a white lab coat and was asked to maintain a consistent hairstyle (whether down or pulled back). Although we acknowledge that not every condition experienced by a consumer in the real world is covered by these specifications, this environment was chosen as the standard because the appearance of the diamonds seems to be most fairly represented without exaggerating or deemphasizing the critical pattern elements.

Trade observations were gathered for several years at the gem shows in Tucson, Arizona, from diamond buyers, sellers, cutters, and appraisers, along with several non-jewelry trade individuals representing consumers. Trade observers took surveys about outline appeal, where each survey page displayed computer-generated outlines for oval, pear, or marquise. Both L:W and outline variations were included, and participants scored each outline from 1 (strongly disliked) to 10 (strongly liked). In 2013, 69 participants were instructed to arrange each set of six diamonds in order from most appealing to least appealing, first evaluating outline and a second time considering only face-up appearance. In 2015, 43 participants provided their comments as they observed reference diamonds of various shapes, including seven ovals, seven marquises, and nine pear shapes (the Tucson set is denoted by T in table 1). These observers evaluated face-up appearance, outline appeal, and durability factors on a scale from Excellent to Poor. Then the observers were asked whether specific features affected their decisions.

In 2014 and 2015, we took the same sets of six diamonds to Antwerp, Hong Kong, Mumbai, Tel Aviv, and New York and gathered observations and comments from 166 participants, all active as diamond buyers, sellers, or cutters. These observers evaluated each stone in a group of six diamonds of a particular shape on a scale from Excellent to Poor and made comments on specific appearance aspects. Most of these observers looked at all 36 oval, pear, and marquise diamonds.

Additional visual observations were gathered from several teams of GIA staff (approximately 30 staff members over three years) adept at assessing outline and appearance details in fancy shapes. Initially, these internal observers worked with the reference sets, forging consensus and refining the relative ranking within the sets. Next, they used the reference stones while observing diamonds submitted for grading reports in sizes from 0.60 to 2.20 ct, evaluating various appearance aspects. Later, two internal teams, one in Carlsbad and one in Las Vegas, observed 683 ovals, 430 pears, and 308 marquise shapes for overall appearance.

Our most recent work with one internal team (2023) has examined appearance details for more client diamonds—approximately 350 ovals, 230 pears, and 90 marquise shapes. Each diamond was observed in the tray, placed in both vertical and horizontal positions. The following were evaluated on scales with either four or five intervals: brightness; girdle reflection; appeal and boldness of the contrast pattern and the symmetry of that pattern; windowing or leakage; size of any bow tie along the stone’s length and width; and the total amount of crushed ice, its sparkle size, definition, and appearance appeal. For some properties, that scale ranged from Excellent to Poor; for others, it ranged from None to Obvious. The contrast pattern was evaluated from Dispersed to Concentrated, and the amount of crushed ice ranged from 0% to 100%. Bow tie patterns were evaluated from Short to Long in one direction and Thin to Wide in the other direction. Crushed ice sparkle size ranged from Tiny to Large.

Direct observation data is presented in histograms by evaluation category. Groups of observations (for a single diamond) were analyzed for their mean and consensus values. Consensus, abbreviated as Cns(x), is a statistical measure developed specifically for ordinal data with arbitrarily assigned number values (Tastle and Wierman, 2007), such as choosing a grade category for an observed diamond. Unlike standard deviation, consensus assesses the frequency distribution of responses to derive a useful measure of agreement (or disagreement) among those responses. Consensus varies from 0 (complete disagreement) to 1 (complete agreement) and indicates the amount of spread among the responses—whether there are many or few of them.

Diamonds were photographed with a Vision360 B2C MiNi system, with lighting conditions comparable to those at the floor of a DiamondDock viewing cabinet. In addition, virtual diamond images have been created using a proprietary configuration with OctaneRender that models many details of the observing environment: the DiamondDock and its lighting, background room lighting, the observer’s position, and even the texture and color of the tray (again, see figure 3). Our observation team compared such virtual images created from the wireframes of the research diamonds to the actual diamonds in the DiamondDock and found the images and diamonds to be nearly identical.

Virtual facet pattern maps (of secondary reflections) were calculated using typical ray-tracing techniques for our reference diamonds and for the 670 recently observed diamonds. These maps independently display a diamond’s face-up visual pattern compared to photographic imaging. They are color-coded to show the size of virtual facets, ranging from light blue (smallest) to dark blue (largest). Figure 4 compares two such maps with virtual images of the diamonds they represent.

RESULTS

An important first step before conducting visual evaluation of fancy shapes was to assemble appropriate sets of oval-, pear-, and marquise-shaped diamonds that displayed a range of visual appearances. Arranging those sets was not difficult, but transforming them into a useful reference set for the evaluation of appearance and outline shape was a more challenging and iterative process. Selected results are presented here, and more comprehensive sets of results can be found in the Data Repository at the bottom of this page.

Table 2 shows the results from the observations in 2013 of face-up appearance for sets A and B in the three shapes. The stones are listed in the rank order for each set according to the internal observing team, next to the ranking derived from the averaged (mean) Tucson observations. Even for oval set B and pear set A, where this order is the same, the consensus values below 0.8 demonstrate the broad spread of opinions among observers. A notable exception is RD137, a marquise that nearly all observers ranked as Poor in appearance. In contrast, pear shape RD131 has a consensus of 0.36—half the observers thought it was Poor, but the other half were distributed from Fair to Excellent.

The outline evaluations (table 3) also show medium to small consensus values for most stones. Again, the reference diamonds are listed according to the ranking of the GIA internal team for outline appeal and can be compared to the mean values from the Tucson observations. (Note that this outline order is different from the appearance order for the same stones within each set.) Pear shapes RD093, RD127, and RD128 (in set A) have higher consensus values for outline evaluation, and oval RD103 has the lowest value. Generally, observers showed considerable variation regarding which outlines were acceptable, let alone attractive. Outline surveys showed similar broad opinions, with some notable differences between what participants liked and outlines that are found in the market (figure 5).

In 2014 and 2015, we took these sets of reference stones to major diamond-cutting centers worldwide and gathered trade observations and comments (see Materials and Methods). The primary goal in collecting such data was to capture any regional differences between the centers. We found substantial agreement for some diamonds, whether the appearance was pleasing or deficient, and marked disagreement for others. Figures 6, 7, and 8 show virtual images of some of the observed reference diamonds paired with histograms of observer appearance evaluations from different locations.

For the ovals in figure 6, observers from all locations agreed well on the high evaluation of RD092, which had minimal windowing, a mixture of virtual facet sizes, and no strong bow tie. RD120, with rather large windowing and low contrast in the center of the stone, was generally disliked, with less agreement about which lower evaluation it should receive. The remaining three diamonds showed a spread of opinions. RD103 and RD110 both received many evaluations of Very Good to Good, but RD103, with a radiating pattern in the table and scattered crushed ice elsewhere, received more high evaluations in all locations but New York; RD110, with heavy concentrations of tiny crushed ice in the wings and regions of contrast under the table, was viewed more favorably in India. Opinions of RD176, which had tiny crushed ice throughout and no sizeable dark regions of contrast, showed considerable variation between locations.

The five pear shapes in figure 7 have general agreement among observers for three diamonds and a wider spread of opinions for the other two. RD135, with a radiating center pattern and a variety of crushed ice zones, was well regarded by most and had a fairly consistent distribution of opinions across all locations. RD127 shows a similar consistency in the distribution but was preferred less overall than RD135. RD130, with significant windows under the table, was strongly disliked by most observers, but a few people in each location gave this stone a higher evaluation. The evaluation distributions of RD131 and RD123 show a strikingly broad range of overall opinions within and between different locations. RD131, with well-distributed crushed ice, was seen more favorably in Antwerp and Hong Kong by a percentage of the observers, though the absolute numbers of people were small. RD123 was seen less favorably in Israel, where 93% of observers gave it a lower evaluation.

Figure 8 shows observations for five marquise diamonds, and geographic differences in evaluation are noticeable for all of them. These differences are most pronounced for RD100, rated lower in Hong Kong and Israel, and RD141, rated higher in Hong Kong and India. Lower values of consensus were found for both bimodal distributions and those with broad spread.

In 2015, additional trade comments and evaluations of appearance and outline were gathered in Tucson. The comments echoed those from international observers and reminded us that an overall cut grading system should address design and craftsmanship issues, as well as appearance. Most trade observers disparaged prominent bow tie patterns and outlines with very large L:W. We also recorded many negative comments about inconsistent girdle thickness and girdle areas that were too thin, even though these factors may not directly affect either appearance or outline shape.

The internal observation team continued refining the RD sets and using them to evaluate diamonds submitted for grading services. These observations began delving into specific pattern aspects (see Materials and Methods), and even the well-trained internal team shows variance among observers, more for some visual properties than for others. Figure 9 displays histograms of consensus values of several appearance aspects for the hundreds of observed diamonds. The internal team agreed very strongly about the extent of windowing for most stones, and strongly for brightness evaluations. However, they showed some variance in the evaluation of the strength of bow tie patterns (contrast boldness) and still less agreement regarding the width of those patterns. Evaluation of whether the contrast pattern was concentrated or dispersed produced lower consensus values for ovals than for pears or marquise shapes. Levels of observer agreement for the amount of crushed ice, its definition, and the predominant sparkle size also show marked differences among the three shapes.

As we examined and considered all these observations, a common theme emerged. Diamonds with substantial amounts of crushed ice in their pattern tended to yield less agreement for either overall face-up appearance or some specific aspects of appearance. The 2023 observations motivated us to develop the virtual facet map (described in figure 4) for displaying crushed ice and analyzing how virtual facets of various sizes are distributed across the stone.

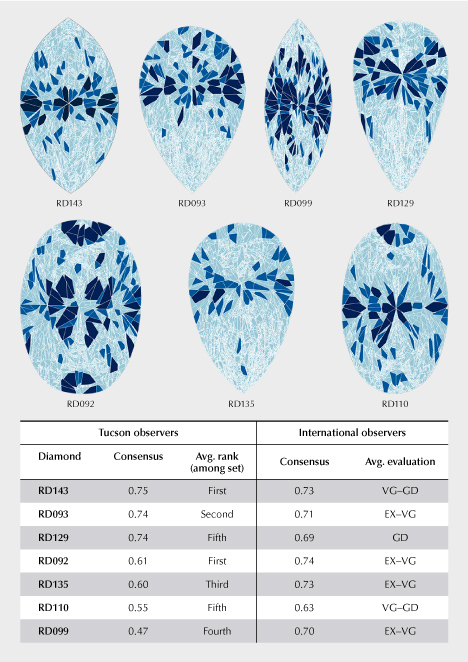
Figure 10 shows virtual facet maps for several reference diamonds observed by both trade and in-house teams; virtual images of these diamonds are found in figures 3, 6, 7, and 8 for comparison. The table in figure 10 gives the evaluations and consensus values from Tucson observers in 2013 and the international observers in 2014/2015. Consensus values for RD143 and RD093 were higher among both groups of observers, with a notable difference in how each group evaluated the appearance of the two diamonds. Marquise RD143 has large virtual facets across the belly with a mixture of large, medium, and small virtual facets filling the points. Pear RD093 has large virtual facets across the head and belly of the stone, plus a few more mixed with medium and small virtual facets in the point.

Observations of oval RD092 and pear shapes RD129 and RD135 yielded strong but different consensus values for appearance between the Tucson group and the international group. Both pear shapes have areas of crushed ice in the head and filling the points. The oval shows two arcs of small virtual facets between the large ones in the belly and at both ends. The virtual facet maps for marquise RD099 and oval RD110 are not symmetrical, another pattern aspect that reduces observer agreement. RD099 has large virtual facets across the belly mixed with medium and small virtual facets toward the points. RD110 has more large virtual facets on the left side and top than on the right side or bottom, as well as large arcs of small virtual facets.

DISCUSSION

A great deal has been discovered about the mechanics of human vision over the last 40 years, but vision psychophysics remains a topic of active research (Murray, 2020). Much of that research is conducted with large visual stimuli—objects more than 10 times larger than gem diamonds—so the industry can learn more from the concepts of such research than from its specific experiments.

Further, people vary substantially in their appreciation of particular aspects of the visual display found in these fancy shapes. A useful cut grading system must make visual sense while acknowledging this variance in personal taste. A fancy cut grading system should support consumer confidence for diamond purchases rather than dictate preferred aesthetics. Human observations are thus essential for sorting out which appearance aspects are evaluated similarly by most observers and which are more subject to individual preference.

Observer preferences vary widely with respect to shape outline (see again table 3 and figure 3). Some observers prefer a narrow range of acceptable shape outlines, while others appreciate a greater variety of outlines, such as those with prominent shoulders, flat wings, and a range of point angles. Observer choices for attractive L:W differ significantly from what is commercially available. Given such a broad range of opinions, reporting L:W and some display of the actual outline shape may be more helpful for both the trade and consumer than setting strict grading limits for these factors. An educational context for the outline variations that typically occur in each shape can help the report user understand how a particular diamond compares to others in the marketplace and assess their personal preference.

When GIA added patterns to evaluate round brilliant appearance (Moses et al., 2004), it was possible to define particular pattern deficiencies and scale them from Poor to Very Good based on observations. Diamonds with proportion combinations that produced none of those deficiencies displayed a range of face-up appearance patterns that were all considered attractive by the majority of the observation team or external observers. These observations show that large windows, especially dark-looking windows, are thoroughly disliked (for example, RD137, RD141, RD130, and RD120). However, an approach limited to winnowing out appearance faults will not account for differences in taste regarding the more complicated patterns seen in oval, pear, and marquise diamonds.

We learned that bow tie patterns that are particularly broad, dark, or persistent (as the stone moves) are generally disliked, but opinions diverge as this pattern becomes narrower, less intense, or changes from dark to light with motion. Some observers expect a bow tie pattern in these shapes, while others prefer a minimal bow tie or none at all. Faceting arrangements with pavilion mains across the stone width (at the belly) often enhance the bow tie. Interestingly, starting in 2014, the GIA laboratory saw a marked shift in the faceting arrangements of oval diamonds submitted for grading services (figure 11). Before that year, more than half of the submissions had faceting arrangements that included pavilion mains across the stone’s width, while 30–40% did not. Since 2014, the percentage of ovals without belly mains has increased to nearly 90%. These arrangements minimize or eliminate the bow tie, and such diamonds sell more easily.

The results showed low consensus values for diamonds with patterns dominated by crushed ice (for example, RD176 from figure 6 and RD131 from figure 7 and table 2). For diamonds with more mixed patterns (containing areas with large virtual facets), some stones show strong agreement for both higher and lower stone evaluations (e.g., RD093 and RD129), while others show only moderate consensus values (see again figure 10). Lack of symmetry in the virtual facet pattern also leads to lower consensus among observers. The virtual facet map provides an analysis of the size and distribution of crushed ice patterns, and future research can examine more closely how the definition and contrast within such patterns contribute to face-up appearance.

Evaluating the cut fitness of each fancy shape—addressing a range of proportions, potential asymmetries, numerous faceting arrangements, and outline variations—is a vast undertaking. We are working toward an objective evaluation of the important aspects of light interplay with a faceted diamond. At the same time, we seek a grading system that accommodates differences in regional and personal taste among the more complex patterns of fancy shapes. Diamonds that observers find similar in bright, fiery, and sparkly appearance can exhibit rather different patterns with more varied overall appeal (figure 12). Tools for analyzing and categorizing those patterns, such as the virtual facet map calculated from a diamond’s measured wireframe file, may provide a valuable addition to a fancy cut grading system.

CONCLUSIONS

A useful cut grading system for fancy shapes must make visual sense for both the trade and consumer. Observations gathered under consistent conditions provide the foundation for developing such a system. Oval-, pear-, and marquise-shaped diamonds display more complex appearance patterns than standard round brilliants, and observation data reveals how that complexity affects the evaluation of appearance.

The elongation of oval, pear, and marquise shapes produces bow ties and areas of crushed ice for many proportion combinations, faceting arrangements, and outline variations. Observations varied regarding the impact of a bow tie on overall appearance, but large, dark, persistent bow ties are a negative factor. Observers disliked diamonds with large windowing but only showed strong agreement when that window was dark. Observer opinion was widely spread for diamonds with substantial areas of crushed ice (small virtual facets), providing evidence of both personal and regional preferences.

Detailed internal observations of pattern elements in these three shapes demonstrated that observers can agree on semiquantitative descriptions of a diamond’s pattern while disagreeing about the appeal of that diamond’s appearance. Matters of taste and personal preference for some pattern elements impact observers’ evaluation of overall appearance. To serve both the trade and the consumer, a cut grading system for fancy-shaped diamonds should accommodate taste differences and provide clarity for understanding them, in addition to evaluating fundamental appearance aspects.

Mathematical representation of fancy shapes, particularly these three fancy shapes, requires more parameters than the six used for the standard round brilliant—far too many for any kind of proportion charts. Instead, an accurate wireframe representation and ray-tracing simulation must serve as the basis for cut grade evaluation and estimation. The virtual facet maps presented here, calculated from such wireframe files, demonstrate some of the advantages of this approach.